A number is said to be a perfect cube if it can be multiplied by itself three times. x must equal y3 if it is a perfect cube of y. As a result, a natural integer, not a fraction, is obtained when we take the cube root of a perfect cube. Therefore, 3x Equals y.

For instance, the number 8 is a perfect cube as 38 = 2. When calculating a cube’s volume, geometry is where cubes are most commonly used (a 3d shape). Learn about the definition, a list of ideal cubes, and examples of applications in this section.
What are Perfect Cubes?
Numbers that are the triple product of the same number are known as perfect cubes. In other words, the value produced by multiplying a whole number by three times itself is a perfect cube. A number that may be expressed as three times the product of another integer is referred to as a perfect cube.
A perfect cube has a cube root that is always a whole number. But regardless of whether a number is an integer or a fraction, we can always find its cube. The cube of 0.6, for instance, is:
0.6 × 0.6 × 0.6 = 0.216
In the section after this one, let’s look at the list of ideal cubes.
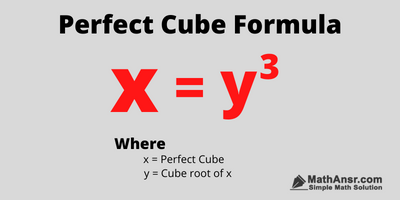
Perfect Cube Formula
x = y3
Perfect Cube List
| Numbers | Multiplied Three times | Cubes |
| 1 | 1× 1× 1 | 1 |
| 2 | 2× 2× 2 | 8 |
| 3 | 3× 3× 3 | 27 |
| 4 | 4× 4× 4 | 64 |
| 5 | 5× 5× 5 | 125 |
| 6 | 6× 6× 6 | 216 |
| 7 | 7× 7× 7 | 343 |
| 8 | 8× 8× 8 | 512 |
| 9 | 9× 9× 9 | 729 |
| 10 | 10× 10× 10 | 1000 |
| 11 | 11× 11× 11 | 1331 |
| 12 | 12× 12× 12 | 1728 |
| 13 | 13× 13× 13 | 2197 |
| 14 | 14× 14× 14 | 2744 |
| 15 | 15× 15× 15 | 3375 |
| 16 | 16× 16× 16 | 4096 |
| 17 | 17× 17× 17 | 4913 |
| 18 | 18× 18× 18 | 5832 |
| 19 | 19× 19× 19 | 6859 |
| 20 | 20× 20× 20 | 8000 |
| 21 | 21× 21× 21 | 9261 |
| 22 | 22× 22× 22 | 10648 |
| 23 | 23× 23× 23 | 12167 |
| 24 | 24× 24× 24 | 13824 |
| 25 | 25× 25× 25 | 15625 |
| 26 | 26× 26× 26 | 17576 |
| 27 | 27× 27× 27 | 19683 |
| 28 | 28× 28× 28 | 21952 |
| 29 | 29× 29× 29 | 24389 |
| 30 | 30× 30× 30 | 27000 |
| 31 | 31× 31× 31 | 29791 |
| 32 | 32× 32× 32 | 32768 |
| 33 | 33× 33× 33 | 35937 |
| 34 | 34× 34× 34 | 39304 |
| 35 | 35× 35× 35 | 42875 |
| 36 | 36× 36× 36 | 46656 |
| 37 | 37× 37× 37 | 50653 |
| 38 | 38× 38× 38 | 54872 |
| 39 | 39× 39× 39 | 59319 |
| 40 | 40× 40× 40 | 64000 |
| 41 | 41× 41× 41 | 68921 |
| 42 | 42× 42× 42 | 74088 |
| 43 | 43× 43× 43 | 79507 |
| 44 | 44× 44× 44 | 85184 |
| 45 | 45× 45× 45 | 91125 |
| 46 | 46× 46× 46 | 97336 |
| 47 | 47× 47× 47 | 103823 |
| 48 | 48× 48× 48 | 110592 |
| 49 | 49× 49× 49 | 117649 |
| 50 | 50× 50× 50 | 125000 |
How to Find the Perfect Cube?
The steps listed below can be used to determine whether a number is a perfect cube:
Step 1: Divide the supplied number into prime factors beginning with the smallest prime number (2).
Step 2: After completing the prime factorization, group together every three identical factors.
Step 3: Repeat the step for each set of the same three factors in the group. The provided number is not a perfect cube if any factors are missing or do not fit into a group of three identical factors. The provided number is a perfect cube in all other respects.

FAQ
The result is known as a perfect cube when a natural number is multiplied by itself three times.
Numbers 1, 8, 27, 64, 125, 216, 343, 512, 729, 1000, 1331, 1728, 2197, 2744, 3375, 4096, 4913, 5832, 6859, 8000, 9261, 10648, 12167, 13824, 15625, 17576, 19683, 21952, 24389, 27000 make up the first 30 cube numbers.
50 x 50 x 50 = 125000 is the perfect cube of 50.

